Groupoids in Mathematics
 |
 |
 |
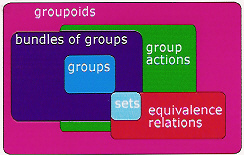
The place of groupoids. |
Union with many base points. |
I=π1([0,1],{0,1}) has two objects 0,1 and 4 arrows |
Groupoids in Mathematics.
There are two opposing views on the place of groupoids in mathematics. One of these, expressed in R. Crowell and R.H. Fox `Introduction to knot theory' [CF], is that a few definitions `like that of a group, or a topological space, have a fundamental importance for the whole of mathematics that can hardly be exaggerated. Others are more in the nature of convenient, and often highly specialised, labels which serve principally to pigeonhole ideas. As far as this book is concerned, the notions of category and groupoid belong in this latter class. It is an interesting curiosity that they provide a convenient systematisation of the ideas involved in developing the fundamental group.' (Fox in his review of [B-vKT] took me to task for quoting the above `out of context'.) The other view is that the natural concept is that of groupoid, of which `group' is a special and useful but not always convenient case; and that systematisations, when convenient, gradually come to show their aid to understanding, and their underlying power. What is clear is that sociologically speaking, and at this date, groups are more important than groupoids.
The fact that directed graphs form the underlying geometry of groupoids enables the latter to combine algebra and geometry, thus avoiding the dichotomy between these two areas suggested in [A]. Indeed the determination of an apparent dichotomy, of an anomaly, can usefully be taken as evidence that the mathematics should be developed to a new form. The combination of algebra and geometry is one of the advantages of what can be called `combinatorial groupoid theory', see Chapter 8 of [B], a natural extension of the standard area of combinatorial group theory. The article [A] also does not mention groupoids, which give power to noncommutative geometry, nor categories, a great unifying aspect of mathematics in the 20th century, with its power of analogy and comparison.
The sensible approach is to say that you use groupoids when convenient, and not otherwise -- just as one sometimes uses matrices and other times linear mappings. Experimentation, analysis and unprejudiced evaluation will help to decide the mathematical value of the groupoid approach. History, and you, dear reader, will decide the outcome! A further point is that the objects (vertices) of a groupoid give a kind of spatial component to group theory and this enables a kind of patching stucture for groupoids not available for groups: see [BBMP]. Again there is a theory of local subgroupoids, [BIM], generalising local equivalence relations.It has recently (August, 2008) struck me how much mathematics has come for me from the initial desire in 1965 to try and find a `better' derivation and exposition of the fundamental group of the circle, and which led me to the use of non abelian cohomology and then of groupoids! What should one make of this, methodologically speaking? Clearly a research plan stating this as an aim in 1965 would have got a rasberry, and indeed the work on higher dimensional van Kampen theorems got rasberries from a variety of UK research panels into the 1990s, whose members seemed unable to distinguish between `mainstream' and `cutting edge'. Also, in terms of methodology I refer to advice of Dirac; there also still seems room for childish questions, in the sense of Grothendieck.
See also this discussion of Groups and groupoids on mathoverflow.
From groups to groupoid: (RB:1987)s (pdf) This file has been revised 2 April, 2012, to use hyperref for the bibliography.Groupoids: relating internal and external symmetry (Alan Weinstein, 1996)
`Three themes in the work of Charles Ehresmann: local-to-global; groupoids; higher dimensions' (pdf) (RB: 2007),
and also the books
Topology and Groupoids (RB: 2006) and
Categories and Groupoids (e-version) (Philip Higgins: 1971, 2005)
Groupoids and crossed objects in algebraic topology pdf revised 2009
My preprint page has relevant presentations on the use of groupoids and higher groupoids.
Nonabelian Algebraic Topology: filtered spaces, crossed complexes, cubical homotopy groupoids Link to Review in Jahresber. Deutsch. Math.-Verein. 114 (2012), 177 - 182 . "Nonabelian phenomena play as well a major role in algebraic geometry (Brauer-Severi varieties, Teichmüller groupoid, etc., to list a few instances). It may well be that, in the future, the ideas presented in the book contribute to some of the many open questions in these areas."
A groupoid can be described as a set G of arrows, a set O of objects, two functions s,t: G →O called the source and target functions, and a composition function xy defined for those arrows x,y such that t(x) = s(y), with s(xy) = s(x), t(xy) = t(y). We then impose axioms of a left identity and a right identity for each arrow x, an inverse x-1 for each x, such that s(x-1) = t(x), t(x-1) = s(x), and finally associativity. (Those who know category theory can say simply that a groupoid is a small category in which every morphism is an isomorphism.) Thus a group may be considered as a groupoid with one object.
The extension from groups to groupoids starts in a formal sense with the desire to describe reversible processes which may traverse a number of states. So the group theory idea is, say, to consider a variety of journeys from say Bangor back to Bangor, whereas in groupoid theory one considers journeys between various cities in the UK, and notes that journeys can be composed if and only if the starting point of one journey is the end point of the previous one. This naive viewpoint gives rise to the heretical suggestion that the natural concept is that of groupoid rather than that of group! In fact the groupoid idea is forced when one tries to structure a journey, i.e. to list the cities travelled.
It is interesting in this respect to note the view of Connes that Heisenberg discovered quantum mechanics by considering the groupoid of quantum transitions of the hydrogen spectrum rather than the group of symmetry.
It is worth explaining how the formal definition of groupoid arose. It dates from Brandt's attempts to extend to quaternary forms Gauss' work on the composition of binary quadratic forms, which has a strong place in his famous work Disquitiones Arithmeticae. Bourbaki cites this latter composition as an influential early example of a composition law which arose not from numbers, even taken in a broad sense, but from distant analogues. Brandt found that each quaternary quadratic form of a given norm had a left unit and a right unit, and that two forms were composable if and only if the left unit of one was the right unit of the other. This led to his 1926 paper on groupoids. (A modern account of the work on composition of forms is given by Kneser et al. [KOKPS].) Groupoids were then used in the theory of orders of algebras: see the details given in an entry in the Springer Encyclopedia. Curiously, groupoids did not form an example in Eilenberg and Mac Lane's basic 1945 paper on category theory.
The usual idea is to consider fundamental groups and `change of base point'; this is like listing return journeys from railways stations, as well as methods of changing from one set of return journeys to another! It is clearly bizarre!
The fundamental groupoid appears in Reidemeister's 1932 book on topology [R] as the edge path groupoid, and for handling isomorphisms of a family of structures. The fundamental groupoid π1(X) of a space X was well known by the 1950's. It consists of homotopy classes rel end points of paths in X, with the usual composition. The fundamental groupoid π1(X,A) of a space X on a set A of base points was introduced in [B-vKT]. Its use extends the validity of the van Kampen theorem, to allow the computation of the fundamental group of spaces which are the union of not necessarily connected subspaces (see diagram at the top of this page). This extended theorem is applied in [B], for example to prove the Jordan Curve Theorem. This book seems to be the only topology text in English to consider π1(X,A) with A having more than one point, and not equal to X. A form of the van Kampen theorem for arbitrary unions of open sets, with a condition that A meets each 3-fold intersection of elements of the cover, is proved in [BR]. For more discussion on the background to the van Kampen Theorem, see the pdf file Groups, groupoids and higher groupoids in algebraic topology . Here is also a complete proof of the pushout version of the van Kampen theorem, taken with modifications but with the pictures from `Nonabelian algebraic topology'.
One should take a hard line with those who suggest a single base point version will do. Thus the path connected space X may be the union of 23 path components whose various three fold intersections have 123 path components. So one needs 123 base points at least! Even more complicated situations arise in applying groupoids to combinatorial group theory. The van Kampen theorem enables the transition from topology to algebra; to obtain information on the vertex groups, i.e. on fundamental groups, one then has to apply standard techniques including choosing trees in components of graphs, techniques which together may be seen as a part of `combinatorial groupoid theory'. This area of techniques is studied and applied in the books [B], [H3] listed below.
If one accepts that all of 1-dimensional homotopy theory is better expressed using groupoids rather than groups, then it is natural to consider the possibilities for the role of groupoids in higher homotopy theory. One key is that whereas group objects in groups are abelian groups, group objects in gropoids are equivalent to J.H.C. Whitehead's crossed modules, and n-fold groupoids are extrememly complicated. This idea of obtaining precise homotopical information by using a bigger structure than that which is traditional is pursued into higher dimensions by using higher homotopy groupoids, though the construction of these is not straightforward, and higher dimensional group theory.
That the first homology of a connected space is the fundamental group made abelian has a generalisation: let A be a discrete subspace of X such that A meets each pathcomponent of X. Then the fundamental groupoid π1(X,A) made abelian is H1(X,A). (The abelianisation of a groupoid is the universal object for morphisms of the groupoid to abelian groups.)
Note that Henry Whitehead observed that a reason for not restricting to one vertex CW-complexes was the need to accomodate covering spaces into the theory. A convenient model for covering maps of spaces is covering morphisms of groupoids.
Operations on higher homotopy groups
If one considers higher homotopy groups πn(X;A) where A is here a set of base points then it is almost inevitable to consider this as a module over the groupoid π1(X,A). It is interesting to speculate what might be higher loop space theory, or `little cubes operads', if you allow a set of base points.
Higgins in [H4] gives an account of normal forms for the fundamental groupoid of a graph of groups: this construction avoids the inelegant but common use of choice of a tree and/or base point. This work is developed into a GAP programme in [Moore], which uses a kind of distributed computing (one machine at the start of every edge). This thesis also develops graphs of groupoids.
Philip Higgins came to his view on the utility of groupoids from reading the account of Hilton, P.J. and Wylie, S., Homology theory, Cambridge Univ. Press, 1960, on covering spaces, and deciding this was mainly groupoid theory.
He then showed the utility of using presentations of groupoids for applications to groups (see also [H1,2,3]). Basically, he showed that some of the topological versions of proofs of classical subgroup theorems (Nielsen-Schreier, Kurosch, Grushko, ...) could be given an analogous algebraic proof using the notion of covering morphism of groupoids, and indeed he considerably generalised Grushko's theorem (see the comments and references in [Bra] below). Note also that covering morphisms of groupoids were defined altough with different terminology in [S].
Indeed the neatest view of covering space theory seems to me the statement that the fundamental groupoid functor gives (for a locally nice space X) an equivalence of categories from the category of covering spaces over X to the category of covering morphisms of the groupoid π1(X).
This is the view taken in the 1968, 1988 and current (2006) editions of the book "Topology and Groupoids", [B]. The 1988 edition is referred to by Peter May in his book "A Concise Course in Algebraic Topology", as "idiosyncratic"; but in fact he partially develops, without reference, an account of covering maps via covering morphisms of groupoids, and gives a use of groupoids in the proof of the van Kampen Theorem, but only to get the fundamental group, and without giving the most general result, as in [BR].
The paper [Braz] develops a notion of "semicoverings" which are better for wild spaces than covering maps. In particular, semicoverings satisfy the "2 out of 3 rule": if two of the maps of a composition pqr are semicovering maps of spaces, then so is the third.
This seems to be the only text on topology which uses the notion of fibration of groupoids ; it is relevant to covering morphisms of groupoids, cofibrations of spaces, and orbit groupoids.
Nielsen and Reidemeister Fixed Point Theory
For an account of applications of fibrations and coverings of groupoids in this area, see this recent paper by Philip I. Heath.
Let us write Gpd for the category of groupoids and morphisms. It contains the category of groups as a full subcategory, but has several nice features not available for groups.
One is that Gpd is cartesian closed. So if H,K are groupoids one can form a groupoid GPD(H,K) such that if G also is a groupoid then there is a natural equivalence
Other features of the category Gpd are the variety of types of morphisms, namely quotient morphisms, retractions, covering morphisms, fibrations, universal morphisms, in contrast to the epimorphisms and monomorphisms of group theory.
In effect, groupoids add to groups the spatial notion of `place', and give a more general perspective than groups on the notion of invertible operation. This becomes clear with the construction of a groupoid from a given groupoid G and a function from Ob(G) which is explained in [H3] and given a wider perpective in [B].
Invertible operations also occur in the theory of inverse semigroups, and there are interesting relations beteen these and ordered groupoids. (Do a web search!)
The category Gpd also has a unit interval object I, namely the groupoid with two objects 0,1 and exactly one arrow 0 → 1. This apparently `trivial' groupoid allows for a useful homotopy theory for groupoids, and so analogies between groupoids and spaces.
If a group G operates on a space X then it also operates on the fundamental groupoid π1X. This leads to the result (RB and P.J. Higgins, 1986) the fundamental groupoid of the quotient of a Hausdorff space by a discontinuous action of a discrete group is the orbit groupoid of the induced action. This allows the calculation of the fundamental groups of some orbit spaces, such as that of the symmetric square of a space. Full details are in Chapter 11 of [B], and are also available in the draft arxiv/math/0212271. I have not seen any result elsewhere in print as strong as this one.
Ross Geoghegan in his 1986 review (MR0760769) of two papers by M.A. Armstrong on the fundamental groups of orbit spaces wrote: "These two papers show which parts of elementary covering space theory carry over from the free to the nonfree case. This is the kind of basic material that ought to have been in standard textbooks on fundamental groups for the last fifty years." At present, to my knowledge, "Topology and Groupoids" is the only topology text to cover such results.
The extension of Galois theory initiated by Grothendieck has required Galois groupoids. For an account, see [BJ].
Groupoidification
This is a form of categorisation in which vector spaces are replaced by groupoids and linear operators by spans of groupoids. See for example [BHW].
For more information on groupoids, see the survey papers listed above, and in the references below, for example [Connes]. Also, a web search on `groupoids' in any one of: analysis; algebra; geometry; physics; computer science; yields thousands of `hits' in each.
There is the important notion of `internal groupoid in a category'; major examples are groupoids in the categories of: directed graphs; simplicial sets; topological spaces; smooth manifolds (Lie groupoids); groups (these are equivalent to crossed modules); rings without identity; Lie algebras; etc.) .
The book [C] discusses the mathematical establishment's long disagreement with the view that groupoids are a sensible generalisation of groups. For further discussion of the notion of paradigm change, see the work of Thomas Kuhn on `The structure of scientific revolutions' (here is just one link on this). Note also the views of Einstein (1915) on the necessity for analysing anew familiar concepts.
There is a considerable literature on the convolution algebra of discrete and measured groupoids, a subject now known as Noncommutative Geometry; see also [Connes]. The origins of this go back to the work of G.W. Mackey on ergodic groupoids (see [M] below), and a meeting with Mackey in 1967 encouraged me to develop the work on groupoids.
The conference proceedings [KPRW] in 2005 gives an account of the large contribution of Charles Ehresmann to wide swathes of mathematics, including especially local-to-global problems, and the use of groupoids (Lie groupoids, foliations, holonomy, germs, jets, fibre bundles, ....). See also [P] for relations of Ehresmann's work with the Erlangen Program of Felix Klein.
I have recently found 850 hits on a web search on "braided groupoids". This an indication of the utility of algebraically structured groupoids. This seems one area where the notion of C*-algebra of a groupoid seems difficult to extend. For example, is there an analogue of a C*-algebra for a groupoid object in the category of Lie algebras?
Strict Higher Homotopy Groupoids
A difficult step was the development of higher homotopy groupoids, as strict generalisations of the fundamental groupoid on a set of base points.
The next diagram gives the idea of the fundamental homotopy double groupoid of a triple (X,A,C) of spaces, namely map a square I2 into X with edges going to A and vertices to C, and then take homotopy classes rel vertices of such maps. This gives the homotopy double groupoid of the triple, and the multiple compositions as shown in the right hand array that this allows lead to a proof (published in 1978, [BH78]) of a 2-dimensional van Kampen Theorem. See also What is and what should be `Higher dimensional group theory'? Link to a beamer presentation of a seminar at Liverpool University, December 4, 2009. pdf
 |
 |
Note that the usual definition of second relative homotopy groups involves somewhat arbitrary choices, and compositions in those groups are all on a line.
For consequences of the 2-dimensional van Kampen Theorem, see Part I of the book advertised at Nonabelian algebraic topology, and for a wider discussion see the article Higher Dimensional Group Theory. That title was intended to show the intuition of developing higher order groupoids with something of the same flavour as group theory. This title also led to the generalisation `Higher dimensional algebra'. Here is a quote from [BSurv]: "I like to think that .... a general advance from 1-dimensional to 2- and n-dimensional algebra could become widely significant. This can be put in the more provocative way: n-dimensional phenomena require for their description n-dimensional algebra." Visitors to the n-category cafe will find that the notion of say n-group is accepted as standard. But that site tends to concentrate on weak globular or simplicial structures.The major part of the above book is devoted to the quite natural but more complicated to realise generalisation to filtered spaces of the above construction for triples, and the consequences.
See also the 2014 presentation available from [B-Paris] below.
Comments from Alexander Grothendieck, 12 April, 1983
What you write about Loday's n-Cat-groups makes sense for me and is quite interesting indeed. When you say they capture truncated homotopy types, I guess you mean "pointed 0-connected (truncated) homotopy types". This qualification seems to me an important one - while they are presumably quite adequate for dealing with a number of situations, it is kind of clear to me they are not for a "passe partout" description of homotopy types - both the choice of a base point, and the 0-connectedness assumption, however innocuous they may seem at first sight, seem to me of a very essential nature. To make an analogy, it would be just impossible to work at ease with algebraic varieties, say, if sticking from the outset (as had been customary for a long time) to varieties which are supposed to be connected. Fixing one point, in this respect (which wouldn't have occurred in the context of algebraic geometry) looks still worse, as far as limiting elbow-freedom goes! Also, expressing a pointed 0-connected homotopy type in terms of a group object mimicking the loop space (which isn't a group object strictly speaking), or conversely, interpreting the group object in terms of a pointed "classifying space", is a very inspiring magic indeed - what makes it so inspiring it that it relates objects which are definitively of a very different nature - let's say, "spaces" and "spaces with group law". The magic shouldn't make us forget though in the end that the objects thus related are of different nature, and cannot be confused without causing serious trouble.
See also this mathoverflow question and links from there.
[A] Atiyah, M., `Mathematics in the 20th century', Bull. London Math. Soc., 34, (2002) 1-15.[BHW] Baez,J., Hoffnung, A.E., and Wa;ker, D.W., `Higher Dimensional Algebra VII: Groupoidification', Theory and Applications Categories, 24 No. 18 (2010) 489-553.
[BBMP] Bak, A., Brown, R., Minian, G., and Porter, T., `Global actions, groupoid atlases and applications', J. Homotopy and Related Structures, 1 (2006) 101-167.
[BJ] Borceux, F. and Janelidze, G., Galois theories, Cambridge Studies in Advanced Mathematics, 72, Cambridge University Press, Cambridge, (2001).
[Bra] Braun, G., `A proof of Higgins's conjecture', Bull. Austral. Math. Soc., 70, (2004), 2, 207--212.
[Braz] Brazas, J., `Semicoverings: a generalisation of covering space theory', Homology, Homotopy, and Applications, 14 (2012), No. 1, pp.33-63.
[B-vKT] Brown, R., `Groupoids and van Kampen's theorem', Proc. London Math. Soc. (3), 17 (1967) 385--401, Math Review 36 #3345, R. H. Fox.
[BSurv] Brown, R., "From groups to groupoids: a brief survey", Bull. London Math. Soc., 19 (1987) 113-134.
[B] Brown, R. "Homotopy theory, and change of base for groupoids and multiple groupoids", Applied Categorical Structures, 4 (1996)" 175-193.
[B1] Brown, R., `Groupoids and crossed objects in algebraic topology', Homology, Homotopy and Applications 1 (1999) 1-78.
[B2] Brown, R., `A 1-dimensional Relative Hurewicz Theorem', arXiv Math/AT: 1012.2824 December, 2010, 1-15.
[B] Brown, R., Topology and Groupoids, Booksurge, 2006.[B-Paris] Brown, R.. Intuitions for cubical methods in nonabelian algebraic topology Talk to the workshop on "Constructive Mathematics and Models of Type Theory", IHP, Paris, June 5, 2014. handout version ; full version
[BH78] Brown, R. and Higgins, P.J., ``On the connection between the second relative homotopy groups of some related spaces'', Proc. London Math. Soc. (3) 36 (1978) 193-212. longer first version 15MB
[BHS] R. Brown, P.J. Higgins, R. Sivera, Nonabelian algebraic topology: filtered spaces, crossed complexes, cubical homotopy groupoids, EMS Tracts in Mathematics Vol. 15, 703 pages. (August 2011).web page
[BIM] Brown, R., Icen, I, and Mucuk,O., `Local subgroupoids II: Examples and properties', Topology and its Applications 127 (2003) 393-408.
[BR] Brown, R. and Razak, A. `A van Kampen theorem for unions of non-connected spaces', Archiv. Math. 42 (1984) 85-88. pdf
[Ca] Cayron, R. `Groupoid of orientational variants', Acta Crystallographica, A 62 (2006) 21-40. (open access)
[Connes] Connes, A. `A view of mathematics'
[C] Corfield, D., Towards a Philosophy of Real Mathematics, CUP, (2003).
[CF] Crowell, Richard H.; Fox, Ralph H. Introduction to knot theory. Reprint of the 1963 original. Graduate Texts in Mathematics, No. 57. Springer-Verlag, New York-Heidelberg, 1977. x+182 pp.
[SGP] M. Golubitsky, M. Pivato, I. Stewart. `Symmetry groupoids and patterns of synchrony in coupled cell networks', SIAM J. Appl. Dynam. Sys. 2 (4) (2003) 609-646.
[GS] M. Golubitsky, I. Stewart, `Nonlinear dynamics of networks: the groupoid formalism' , Bull. Amer. Math. Soc. 43 (2006), 305-364
[H1] Higgins, P. J., `Presentations of groupoids, with applications to groups'. Proc. Cambridge Philos. Soc. 60 (1964) 7-20.
[H2] Higgins, P. J., `Grushko's theorem'. J. Algebra 4 (1966) 365-372.
[H3] Higgins, Philip J., Notes on categories and groupoids. Van Nostrand Rienhold Mathematical Studies, No. 32. Van Nostrand Reinhold Co., London-New York-Melbourne, 1971. v+178 pp. Reprinted as a Theory and Applications of Categories Reprint No 7, 2005.
[H4] Higgins, P.J., `The fundamental groupoid of a graph of groups', J. London Math. Soc. (2), 13 (1976) 145--149.
[KOKPS] Kneser, M.; Ojanguren, M.; Knus, M.-A.; Parimala, R.; Sridharan, R., `Composition of quaternary quadratic forms'. Compositio Math. 60 (1986), no. 2, 133-150.
[KPRW] `The mathematical legacy of Charles Ehresmann' conference `Geometry and Topology of Manifolds' Bedlewo 2005, Editors: Jan Kubarski, Jean Pradines, Tomasz Rybicki and Robert Wolak, INSTITUTE OF MATHEMATICS · POLISH ACADEMY OF SCIENCES, BANACH CENTER PUBLICATIONS 76 (2007).
[Mackenzie] Mackenzie, K.C. H., General theory of Lie groupoids and Lie algebroids, London Mathematical Society Lecture Notes 213 CUP (2005).
[M] Mackey, G.W., Ergodic Theory, Group Theory, and Differential Geometry, Proceedings of the National Academy of Sciences of the United States of America, Vol. 50, No. 6 (Dec. 15, 1963), pp. 1184-1191.
[Moore] Moore, E.J., Graphs of groups: word computations and free crossed resolutions, PhD thesis, University of Wales, Bangor, (2001). pdf 114pp.
[P] Pradines, J. In Ehresmann's footsteps: from group geometries to groupoid geometries. (English summary) Geometry and topology of manifolds, 87 - 157, Banach Center Publ., 76, Polish Acad. Sci., Warsaw, 2007.
[R] Reidemeister, K., Einführung die kombinatorische Topologie, Braunschweig, Berlin (1932); reprint Chelsea, New York (1950).
[S] Smith, P.A., `The complex of a group relative to a set of generators. I,II', Ann. of Math. (2), 54 (1951) 371-402, 403-424.
[Wallace] Wallace, R. `Metabolism, reproduction, and chirality: Insights from a groupoid stereochemistry', Preprint, Oct 2008, New York State Psychiatric Institute.
[W] Weinstein, Alan, `Groupoids: unifying internal and external symmetry. A tour through some examples'. in Groupoids in analysis, geometry, and physics (Conference, Boulder, CO, 1999), edited A. Ramsay, J. Renault 1-19, Contemp. Math., 282, Amer. Math. Soc., Providence, RI, 2001.
[Z1] Zivaljevic, Rade T., Combinatorial Groupoids, Cubical Complexes, and the Lovász Conjecture. Discrete and Computational Geometry 41(1): 135-161 (2009)
[Z2] Zivaljevic, Rade T.(SE-SAOS) Groupoids in combinatorics---applications of a theory of local symmetries. Algebraic and geometric combinatorics, 305--324, Contemp. Math., 423, Amer. Math. Soc., Providence, RI, 2006.
Updated February 12, 2018
